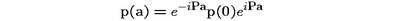Are different forms of relativistic dynamics physically equivalent?

The concept of the form of dynamics was introduced by Dirac in 1949 [1]. Any quantum relativistic system is described by a Hilbert space in which there is a unitary representation of the Poincare group. This representation has 10 Hermitian generators: time translations are generated by the Hamiltonian H, space translations are generated by the vector of the total momentum P, rotations are generated by the vector of the total angular momentum J, and boosts are generated by the vector of boost K. In an interacting theory, some of these generators may be interaction-dependent, while others are interaction-free. The different choices of interacting generators are called different "forms of dynamics", according to Dirac. There is a wide-spread opinion that different forms of dynamics are physically equivalent. Here I would like to show that this opinion is not correct.

First consider the instant form of dynamics in which only the Hamiltonian H and the boost operator K have interaction contributions V and W, respectively

Let us now see how certain observables transform with respect to space translations. Consider the momentum operator p of one particle in the system. Denote p(0) the operator corresponding to the initial reference frame, and p(a) the momentum operator in the reference frame translated by the distance a. Then, basic rules of quantum mechanics tell us that

(1)

In the instant form of dynamics p(0) commutes with P, so particle's momentum is not affected by the translation of the observer. This is exactly what we observe in nature.

Now, let us take up the case of the point form of dynamics. The characteristic property of this form is that the Hamiltonian H and the total momentum operator P contain interaction terms, while J and K are the same as in the non-interacting theory.

If we now apply space translation (1) to the momentum operator of the particle and take into account that normally interaction Z does not commute with p(0), we will see that space translations change the observed values of the particle's momentum. In other words, the measurement of momentum depends on the distance between the observer and the observed particle. Such a behavior has never been seen in experiment. Therefore, the point form of dynamics is ruled out.

It is true that the S-matrix does not depend on which form of dynamics is used in calculations. This explains successful applications of different forms of dynamics (e.g., the point form and, especially, the front form) in calculations of scattering and energies of bound states in nuclear physics. But this is an entirely different matter, and I will discuss it in a different post. The conclusion of this post is: different forms of dynamics are scattering equivalent, but they are not physically equivalent.

[1] P. A. M. Dirac, Forms of relativistic dynamics , Rev. Mod. Phys., 21 (1949), 392.

Is there Minkowski space-time?

The issue of the non-universality and interaction-dependence of Lorentz transformations was discussed here and here . It was shown that in a rigorous quantum relativistic setting, the Lorentz transformations (for the energy-momentum of particles as well as for the position-time of events) depend on the interaction acting in the physical system. The boost transformations of particle observables are sensitive to the presence of other particles around and on interactions with them.

This means that one cannot rigorously introduce the idea of Minkowski space-time. Indeed, this idea works only in the case when all physical systems obey exactly the same boost transformation laws - the Lorentz transformations. Only then one can say that these are not simply transformations of individual particle observables, but global transformations of space-time coordinates (similar to universal and interaction-independent spatial shifts and rotations). This is the story told to us by Einstein's special relativity, and this is an old story that should be changed.

• Q.: Are you saying that particle observables, such as momentum and energy, do not transform according to covariant tensor laws?
• A.: Yes.
• Q.: Then how do they transform when the velocity of the observer changes?
• A.: The exact transformation laws are very close to the usual Lorentz formulas, but they include small corrections that depend on the state of the system of particles and on the interaction acting there. There is no universal formula.
• Q.: Can you say something more definitive?
• A.: Yes, I can. One can perform a rigorous calculation of the slowing down of the decay of fast moving particles and show that Einstein's time dilation formula holds only approximately.
• Q.: But this formula was confirmed in particle decay experiment to a great precision.
• A.: It is true. However, the corrections to the decay laws of moving particles predicted by my approach are several orders of magnitude smaller than the resolution of modern instruments.
• Q.: I find it difficult to believe that the Minkowski space-time picture that served us well for almost 100 years should be abandoned. A huge part of theoretical physics is built upon this formalism.
• A.: Let me give you one more argument. Please compare two kinds of inertial transformations: time translations and boosts. It doesn't look surprising that the result of a time translation is interaction-dependent. The time evolution of an interacting system is quite different from the time evolution of a non-interacting system. Why boosts should be different? In the instant form of Dirac's dynamics, the boost operator (just like the Hamiltonian) is interaction-dependent. Therefore we should expect that boost transformations of observables in the interacting system should be different from the transformations in the non-interacting system. There is a full analogy with the time evolution.
• Q: If we abandon the Minkowski space-time and covariant tensor transformation laws, then there is nothing left from the theory of relativity.
• A: I do not agree. The theory becomes more complicated than Einstein's special relativity with universal space-time. This is true. However, the most important ingredient of any relativistic theory is not affected: the invariance of physical laws with respect to the Poincare group of inertial transformations is strictly maintained. Together with the laws of quantum mechanics, this is sufficient for performing all kinds of quantum relativistic calculations.

More about the (non-)universality of Lorentz transformations

In a previous post we discussed Lorentz transformations in special relativity. We established that there are no a priori reasons why these transformations should be considered exact and universal.

Here is another argument showing that Lorentz transformations actually cannot be exact and universal. Consider any relativistic quantum theory (QFT, for example). In the Hilbert space of the system there should be an unitary representation of the Poincare group with ten generators

(see, for example, [1], eqs. (3.3.18) and (3.3.20)). Note that the presence of the interaction term W in the boost operator K is absolutely required in order for the theory to be relativistically invariant, i.e., in order to maintain the Poincare commutation relations between generators H, P, J, K.

The operator K generates boost transformations of observables. So, if p(0), for example, is the momentum of one particle in the reference frame at rest, then the momentum of this particle in the moving reference frame is given by

(1)


where z is the rapidity of the boost. Now it is easy to see that if in formula (1) we use the non-interacting boost operator (with W=0), then it leads exactly to the familiar Lorentz formula for momentum-energy. So, Lorentz transformations are definitely applicable to non-interacting systems of particles.

However, if in eq. (1) we use the full interacting boost operator K, then the boost transformation is different from the simple linear Lorentz formula, because operator W generally does not commute with p(0). Thus, momentum-energy boost transformations of interacting particles depend on interaction. This demonstrates that universal interaction-independent Lorentz transformations contradict relativity (=the Poincare group properties) and quantum mechanics.

[1] S. Weinberg, The Quantum Theory of Fields vol. 1 (1995)

Are Lorentz transformations exact and universal?

Textbook presentations of special relativity usually start from two Einstein's postulates:

• Postulate 1 (the principle of relativity): All inertial frames of reference are equivalent.
• Postulate 2 (the invariance of the speed of light): The speed of light is the same for all observers and does not depend of the speed of the source.

Then the textbook discusses various physical events usually associated with light pulses. For such events one can use the Postulate 2 and derive the beautiful results of special relativity: the time dilation, the length contraction, the relativity of simultaneity, etc. All these results are summarized in the Lorentz transformations for the time and position of events. All this is fine and good.

The problems begin when one wants to extend the Lorentz transformations and other special-relativistic formulas to events associated not with light pulses, but with systems of interacting particles. How can we do that? There is no Postulate telling us anything about interacting particles. So, when we say that Lorentz transformations apply universally to all events and phenomena in the world, we make a huge leap of faith. Actually, we introduce into special relativity a third Postulate

• Postulate 3 (the universality of Lorentz transformations) The Lorentz transformations of special relativity apply to all events independent on their nature and involved interactions.

Only after this Postulate is introduced, we can notice the suspicious symmetry between spatial and temporal coordinates of events and how they transform to the moving frame of reference. Only then we can follow Einstein and Minkowski and boldly introduce the idea of space-time unification into one 4-dimensional continuum.

The question I would like to ask is this: What is the reason for introducing this third (hidden) Postulate? Yes, I know how well special relativity agrees with experiment. However, it could be possible that one can abandon Postulate 3 and still keep this perfect agreement with experiment. Actually, this is possible. One can construct a theory in which Postulates 1 and 2 are respected, but Postulate 3 is not. In this theory, therefore, there is no place for the 4D Minkowski space-time. In this theory, the Lorentz transformations are violated by a tiny amount that depends on the strength of interaction acting in the physical system. These violations are, in principle, observable. However, they are a few orders of magnitude less than the resolution of modern instruments. More about that in later posts.

What is QFT?

In 1997 Steven Weinberg published a fascinating little article What is Quantum Field Theory, and What Did We Think It Is? On 17 pages he managed to give three totally different definitions of Quantum Field Theory ...and I quote:

1. "The bottomline is that quantum mechanics plus Lorentz invariance plus cluster decomposition implies quantum field theory." This suggests to me that QFT is just a mathematical formalism that allows one to satisfy these three respectable principle. It is not prohibited to look for other formalisms that satisfy the same principles. Weinberg himself says that there is no proof that QFT is the only option.
2. "In its mature form the idea of quantum field theory is that quantum fields are the basic ingredients of the universe, and particles are just bundles of energy and momentum of the fields." Probably I was wrong, and there is no way around fields. Fields rule!
3. "The present educated view of the standard model, and of general relativity, is again that these are leading terms in effective field theories." Translation: quantum field theory is not fundamental. QFT is simply a low-energy approximation to some truly fundamental theory. Needless to say that nobody has a clue what this truly fundamental theory is.

Which of these three statements is (more) correct? "Bottomline", "mature form", or"educated view"? I'll tell you my opinion in another post.

Hello world!

• Q: What is the point of opening this blog?
• A: I would like to discuss here some fundamental problems in theoretical physics.
• Q: Like what?
• A: What is Minkowski space-time? Can one modify quantum mechanics? What are quantum fields? Is renormalization necessary? Is Maxwell's theory an accurate description of classical electrodynamics? What is the meaning of the gauge invariance?
• Q: Isn't it easier to pick up a textbook and find the answers there?
• A: Yes, if you believe that textbooks give you complete and precise answers. I have some doubts about that. I believe there are grey areas and lots of unanswered questions.
• Q: Why do you think these issues are important?
• A: It is not a secret that modern theoretical physics is in crisis. I believe that we cannot move forward without full understanding of foundational questions.
• Q: Crisis?.. What crisis!? Do you know about the Standard Model and Einstein's General Relativity which precisely describe all observable phenomena?
• A: Yes, I know about impressive results of these theories. However, I also know about some flaws and inconsistencies there. I would like to discuss them at this blog.
• Q: Are you going to offer a solution to the problem of quantum gravity?
• A: I have some ideas about that. However, I think, before moving into that territory it is important to reexamine the foundations: Special Relativity, Quantum Mechanics, and their unification.
• Q: What is the point of doing that? Very smart people worked on these issues for more than 100 years. Everything that can be solved and understood is already solved and understood. Special Relativity and Quantum Mechanics are happily united in Quantum Field Theory and, ultimately, in the Standard Model.
• A: Well, if everything is so cool, then why the unification of SM and GR is such an intractable problem? Something should be wrong in our present understanding of Nature. Let us review what has been done in the last 100 years. Maybe we will find some unexplored areas and some new ways of looking at things.
• Q: What do you think about string theory?
• A: No comments.
• Q: What do you think about loop quantum gravity?
• A: No comments.
• Q: What do you think about Axiomatic (Algebraic) QFT?
• A: No comments.